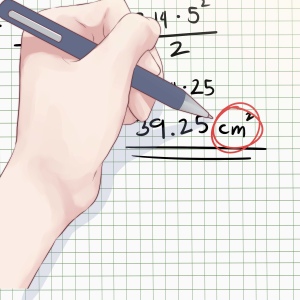Square is a geometric figure, which has four sides of the same length, which are located at an angle of 90 degrees relative to each other. In other words, this is a kind of right rectangle. In some cases, the square is called one of the variants of the rhombus.
The diagonal of the square is a segment crossing the central point of the square and connecting its opposite angles. On one square placed 2 diagonals of the same length.
Calculation of the square of the square, taking into account the length of the diagonal
- The length of the square diagonal is involved in the formula for calculating the square of the square. Denote the length of the diagonal D, and the square S. S \u003d D ^ 2/2.
- The length of the diagonal of the square can be calculated using the Pythagora theorem. Considering the fact that the diagonal of the square is the hypotenus of a rectangular anose-free triangle, we have the following formula for calculating the length of the hypotenuse: A ^ 2 + A ^ 2 \u003d D ^ 2, where A is the length of one side of an equally sided triangle or square. Then D \u003d A√2.
- For example, if you take a diagonal length of a square equal to 4 cm, then its area will be equal to: S \u003d 4 ^ 2/2 \u003d 8 kV. cm.
- If the square is included in the circle, and the length of the diameter of the circle is known, then it is necessary to clarify that the length of the diameter of the circle and the length of the square diagonal is equal to each other. Therefore, in this case, we again go to the calculation of the square of the square through its diagonal.

Calculation of the square of the square, taking into account the length of the side of the square
- From the topic considered above, it follows that when substituting the expression D \u003d A√2 in the formula of counting the square S \u003d D ^ 2/2, we go to the possibility of calculating the square of the square through the length of its side: S \u003d (A√2) ^ 2 / 2, then s \u003d a ^ 2.
- We calculate the length of the side of the square, based on the previously calculated area, equal to 16 cm. A \u003d √S \u003d √8 \u003d 2.83 cm.

Calculation of the square of the square, taking into account the length of the perimeter of the square
- If we know the length of the perimeter of the square, and it is necessary to calculate the area of \u200b\u200bthe figure, then you need to clarify what is the perimeter of the square. The perimeter is the value obtained by summing up all lengths of the side of the geometric shape.
- Denote perimeter P, then p \u003d 4a. Then the length of the side of the square will be equal to A \u003d P / 4. This expression is substituted in the formula of calculating the square of the square S \u003d A ^ 2 and we obtain S \u003d (P / 4) ^ 2, that is, S \u003d p ^ 2/16.
- For example, if the perimeter of the square is 20, then S \u003d 20 ^ 2/16 \u003d 25 kV. cm.