How to find a quadrangle area

When solving the planimetic tasks of the geometry course, a figure with 4 sides is often encountered. Yes, we are talking about a quadrangle. An arbitrary polygon with four angles is less common than its private cases, trapezoids, delto, parallelograms. The last "group" also includes diamonds, rectangles, squares.
Consider what data the figures you need to know to calculate its area.

1
How to find a quadrangle area

Polygon arbitrary

To find its area, you will need a diagonal of the shapes, as well as the angle obtained as the result of their intersection.

S \u003d (d1 * d2 * sinα) / 2,
d1, D2 - diagonal,
α is the angle obtained by intersection.

Polygon in circle

If the specified quadrangle is placed in a circle, the length of the parties is known, the ratio will help in the definition of the polygon area:

S \u003d √ (p - m) (p - k) (p - L) (p - e), p \u003d (m + k + l + e) \u200b\u200b/ 2.
M, K, L, E - His sides.

2
How to find a quadrilateral area - trapezoids

This figure features the presence of parallel 2-sides. To determine the area of \u200b\u200bthis polygon, use these parameters:

If the magnitudes of parallel sides and perpendicular heights carried out to them, the area is calculated using the expression S \u003d ((A + B) * H) / 2,
a and b - grounds,
h - perpendicular height.
Based on the definition of the intermediate line (k \u003d (a + b) / 2)), the previous formula will acquire the following form: S \u003d K * H,
K - line of the middle.
The well-known diagonals of the trapezium and the degree of the corner, formed as a result of their intersection, will also help determine the area of \u200b\u200bthe figure: S \u003d (D1 * D2 * SINβ) / 2,
D1, D2 - diagonal,
β - angle obtained by intersection.
4 sides are given: s \u003d ((m + l) √k ² - ((M - L) ² + K. ²- D. ²)²/ (4 (M - L) ²))/2,
M, L - side parallels,
k, d - side sides.

3
How to find a square of a quadrangle - Deltaida

Polygon-deltoid is characterized by the presence of 2 pairs of equal parties. Calculate the area of \u200b\u200bsuch a quadrilateral is calculated as follows:

The sides of the figure and an angle formed by the sides of different lengths are known:
S \u003d m * l * sinφ,
M, L - Delta side,
φ is the angle between them.
The sides of the shapes and the angles formed by the parties of equal length are known.
S \u003d M. ²* SINα / 2 + L ²* SINβ / 2,
M, L - Delta side,
α, β - angles between equal parties.
The presence of known diagonals also allows you to determine the area of \u200b\u200bthe figure:
S \u003d d1 * d2 / 2,
D1, D2 - Diagonal Deltaida.
If a circle is inscribed in the figure, the knowledge of its radius allows you to calculate the area of \u200b\u200bthe delto: S \u003d (M + L) * R,
M, L - Delta side,
R is a radius in case of inscribed circle.

4
How to find a quadrangle area - a parallelogram

If the convex polygon has 2 pairs of inhabitable sides, then before you - parallelograms.

General expression

To determine the area of \u200b\u200bthis species, the figure will require:

The side of the quadrilateral and height, lowered to it: s \u003d k * h (k),
k - side of the figure,
H (k) - height to her.
The length of both sides having one vertex, and a degree of the corner at a given vertex:
S \u003d l * k * sinφ,
k, L - Polygon sides,
φ is the angle between them.
The diagonals of the figures and an angle obtained as the result of their intersection: S \u003d D1 * D2 * SINβ / 2,
D1, D2 - diagonal,
β - angle - the result of their intersection.

Rhombus

This quadrilateral is a special case of a parallelogram having 4 equal sides. Therefore, the expressions are valid for the parallelogram are true for him. Then

S \u003d k * h (k),
k - side of the figure, h (k) - height to it.
S \u003d K. ²* sinφ,
k is the side of the quadrangle, φ is the angle between the parties.
S \u003d d1 * d2 / 2 (because diagonally shapes when crossing a straight line angle, and sin90 ° \u003d 1),
D1, D2 - diagonal polygon.

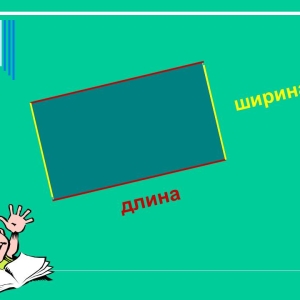
Rectangle

Such a polygon has 2 pairs of equal parties, and the degree of its angles is 90 °. To find its area, the following expressions are valid:

S \u003d K * L,
K, L - sides of the figure.
S \u003d D. ²* SINβ / 2,
D is the diagonal of the quadrangle, β is the angle - the result of their intersection.
S \u003d 2r. ²* sinβ,
R is a radius in the case of the circle described.

Square

In this case, the relationship obtained at the previous stage will acquire the following form (because the sides of this type of rectangle are equal to):