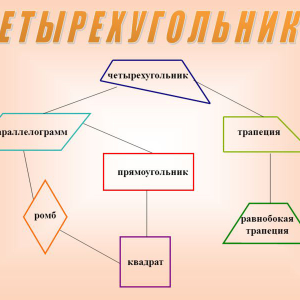
Familiarity with trapezium is happening for the first time when studying the planning rate. Although before that you probably met the items that the form of which coincides with this geometric figure. The quadrilateral is characterized by the fact that only 2 of its four sides are parallel. If you connect the opposite vertices of the figures with segments, we will get it diagonally. How to determine their length? The magnitude of these segments is associated with the angles of the figure, the length of its sides and height.
Diagonal and corners of the trapezium
If you have an arbitrary trapezium with known angles at the base, as well as side sides and base, then the following ratio will help in determining the size of diagonals:
d1 \u003d √a 2 + D. 2 - 2AD * Cosβ,
d2 \u003d √a 2 + C. 2 - 2ac * cosα,
d1, D2 - the desired diagonals,
a - the foundation
C, D - side sides,
β, α - angles lying at the base.
It is based on the cosine theorem, which allows in a triangle to determine the length of the parties using the known values \u200b\u200bof two other sides, as well as the angle lying against the desired side.
Diagonal and sides of the trapezium
- In the presence of all four sides, the shapes for finding its diagonals can use expressions:
d1 \u003d √ D 2 + AB - (A (D 2 - C. 2) / (a-b))
D2 \u003d √ C 2 + AB - (A (C 2 - D. 2) / (A-B)).
- The relationship between the diagonals:
d1 2 + D2. 2 \u003d C. 2 + D. 2 + 2ab,
D1 \u003d √C. 2 + D. 2 + 2AB - D2 2,
D2 \u003d √C. 2 + D. 2 + 2ab - D1 2,
In both the first and second cases:
D1, D2 - the desired diagonals,
a, b - grounds,
C, D - side sides.
Diagonal and height of the trapez
With the known value of one of the bases of the figure or the side, the angle at the lower base, as well as the height of the quadrilateral, with the definition of the lengths of diagonals, there will also not be difficult.
d1 \u003d √h. 2 + (A - H * CTGβ) 2,
D1 \u003d √h. 2 + (B + H * Ctgα) 2,
d1 \u003d √a 2 + D. 2 - 2a √d. 2 - H. 2,
d1 \u003d √h. 2 + (A - H * Ctgα) 2,
D1 \u003d √h. 2 + (B + H * Ctgβ) 2,
d1 \u003d √a 2 + C. 2 - 2a √C. 2 - H. 2,
d1, D2 - the desired diagonals,
a, b - grounds,
β, α - angles lying at the base.
C, D - side sides,
H is the height of the figure.
Diagonal and middle line of trapezium
If the average line is present in the number of the specified values, then with its help you can also calculate the length of the diagonals of the figure. The ratio is true only in cases when sinφ \u003d sin γ.
Because l \u003d d1 * d2 * sinφ / 2h \u003d d1 * d2 * sin γ / 2h,
d1 \u003d 2hl / d2 * sinφ \u003d 2HL / D2 * SIN γ,
d2 \u003d 2hl / d1 * sinφ \u003d 2hl / d1 * sin γ,
d1, D2 - the desired diagonals,
φ, γ - angles between them,
h - the height of the figure,
L - its middle line.
Figure equaloboca
If, according to the terms of the task, the trapezium has equal side sides, the expressions for finding the diagonals of the figure are transformed with the fact that C \u003d D:
d1 \u003d D2 \u003d √C 2 + AB,
d1 \u003d d2 \u003d √a 2 + C. 2 - 2ac * cosα,
d1 \u003d d2 \u003d √a 2 + C. 2 + 2ac * cosβ,
D1 \u003d D2 \u003d √B 2 + C. 2 - 2bc * cosβ,
D1 \u003d D2 \u003d √B 2 + C. 2 + 2BC * COSα,
d1 \u003d d2 \u003d √h 2 + L. 2,
d1 \u003d d2 \u003d √h 2 + (A + B) 2/4,
d1 \u003d d2 \u003d √h * (a + b) / sinφ \u003d √2s / sinφ \u003d √2lh / sinφ (sinφ \u003d sin γ),
d1, D2 - the desired diagonals,
φ, γ - angles between them,
h - the height of the figure,
S - Area,
a, b - base (a \u003cb),
C - side,
L - middle line.